T
Traveler 25042
EVENTUALLY they will come around, I tell you.
Once people start reframing their perspectives from "This is one universe that we may or may not be in a multiverse that we can't detect or interact with known science" to "We live in a multiverse that is in constant interaction. The ways and methods to detect it are already there, but we haven't known how to interpret it correctly."
Because check this out. This is another one for Professor @RainmanTime to get around to it. I mean this respectfully.
What does time travel look like? Y'know, mathematically...
I can tell you the grandfather paradox looks like this. Looks kinda familiar right? This is an easy one.

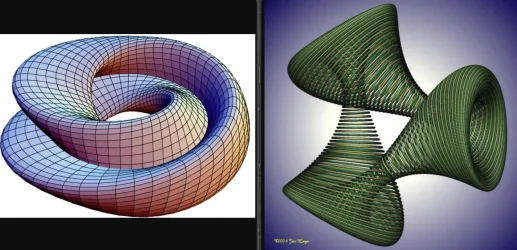
But like....it can also look like this. Really there's infinite ways to do it. The geodesics are mostly just toroids.

Or this or this. The one on the right is a triple paradox.
You can get real nutty with it, and the multiverse doesn't give 2 cents as long as you're not trying to break the universal constants.
Alright so what's the math look like then? Well, I'm going to give it to you in the Multiversal Mechanics notation, and then if someone wishes, they can do the substitutions for General Relativity and/or Quantum Mechanics.
I will denote a paradox with the letter X.
Any given paradox X is equal to the intersection (∩) between two universes (P1 and P2)
So then, X = P1 ∩ P2
I already explained how to calculate the convergence in an earlier post, so I'm not going to repeat most of it here.
The big takeaway is that as a universe converges paradoxically, the union of flux points (Y) between the two universes approaches null.
If the paradoxical intersection of universes is ∅, it means a stable convergence (aka paradox) has completed somewhere.
Multiversal paradoxes are measurable and surprisingly common.
Not to get too on the nose with the pop culture reference, but the universe really is more like a big ball of wibbly, wobbly, timey wimey stuff lol
It's all totally natural too. That is, until you start breaking universal constants. HOO BOY does that become complicated! Math nerds will have orgasms. M theorists will have nightmares. Perhaps I will divulge go into more depth on that another day.
Once people start reframing their perspectives from "This is one universe that we may or may not be in a multiverse that we can't detect or interact with known science" to "We live in a multiverse that is in constant interaction. The ways and methods to detect it are already there, but we haven't known how to interpret it correctly."
Because check this out. This is another one for Professor @RainmanTime to get around to it. I mean this respectfully.
What does time travel look like? Y'know, mathematically...
I can tell you the grandfather paradox looks like this. Looks kinda familiar right? This is an easy one.

But like....it can also look like this. Really there's infinite ways to do it. The geodesics are mostly just toroids.

Or this or this. The one on the right is a triple paradox.
You can get real nutty with it, and the multiverse doesn't give 2 cents as long as you're not trying to break the universal constants.

Alright so what's the math look like then? Well, I'm going to give it to you in the Multiversal Mechanics notation, and then if someone wishes, they can do the substitutions for General Relativity and/or Quantum Mechanics.
I will denote a paradox with the letter X.
Any given paradox X is equal to the intersection (∩) between two universes (P1 and P2)
So then, X = P1 ∩ P2
I already explained how to calculate the convergence in an earlier post, so I'm not going to repeat most of it here.
The big takeaway is that as a universe converges paradoxically, the union of flux points (Y) between the two universes approaches null.
If the paradoxical intersection of universes is ∅, it means a stable convergence (aka paradox) has completed somewhere.
Multiversal paradoxes are measurable and surprisingly common.
Not to get too on the nose with the pop culture reference, but the universe really is more like a big ball of wibbly, wobbly, timey wimey stuff lol
It's all totally natural too. That is, until you start breaking universal constants. HOO BOY does that become complicated! Math nerds will have orgasms. M theorists will have nightmares. Perhaps I will divulge go into more depth on that another day.
